

Second-harmonic generation in one-dimensional photonic crystals from porous silica has been experimentally studied by the scientists and post-graduate students of the MSU Faculty of Physics (associate prof. T.V. Murzina, prof. B.I. Mantsyzov, assist. S.Y. Svyakhovskiy, research assist. A.I. Maydikovskiy, post-graduate stud. D.A. Kopylov, and post-grad. stud. V.B. Novikov at the Department of Quantum Electronics and General Physics).
The frequency conversion of light is a problem of greatimportance in laser physics. Using periodic dielectric structuresallows one to achieve conversion at a high level of efficiency.Photonic crystals (PC) are dielectric structures whosediffraction value is modulated on the scale of the wavelengthof light. In such objects, phase synchronism – necessary for second-harmonic (SH) generation – is achieved due to the peculiarities of light dispersion near the edges of photonic forbidden zones. The efficiency of non-linear conversion can be enhanced by the effects of localizing the electromagnetic field and reducing the group velocity of light. The properties of one-dimensional photonic crystals are usually studied in terms of Bragg geometry, and are considered in a wide range of investigations. Since PC consist of several hundreds of layers and have sufficient width, it is possible to studynon-linear processes in PC applying Laue geometry as well,which has not yet been carried out yet.
It is worth noting that, generally, there are no forbidden zones in Laue geometry. Radiation within PC is localized in alternating layers and is propagated between the modes: the Borrmann mode (B) and the anti-Borrmann mode (aB), corresponding to layers of low and high values of diffraction. Second-harmonic generation for six types of synchronism between excitation modes and SH modes was theoretically predicted by A.P. Sukhorukov.
The non-linear properties of one-dimensional PC were studied by a group of scientists from the MSU Faculty of Physics using one-dimensional photonic crystals based on porous silica created at the faculty. Optical non-linearity was created in them in two ways: by introducing ferroelectric salt (sodium nitrite) into the pores with the help of a partially thermally oxidized sample, in which non-linearity is created by surfaces of silicon nanocrystallites.
In the first case, the condition for synchronism was chosen theoretically by varying relative layer widths. A photonic crystal was made in accordance with the parameters defined. The experiment was carried out for phase synchronism between anti-Borrmann excitation modes and second-harmonic modes. Indicatrices of second-harmonic intensity were considered depending on the incidence angle of radiation, wavelength and light polarization. Second-harmonic generation was revealed to occur near the Bragg incidence angle and form two sharp peaks in the direction of the zero and first diffraction orders. One of the peaks is shown on Fig.1.
In the second case, in photonic crystals made of partially oxidized porous silica, phase synchronisms between anti- Borrmann excitation modes and second-harmonic modes, as well as between anti-Borrmann and Borrmann excitation modes and Borrmann second-harmonic modes were detected in full accordance with the previously developed theory.
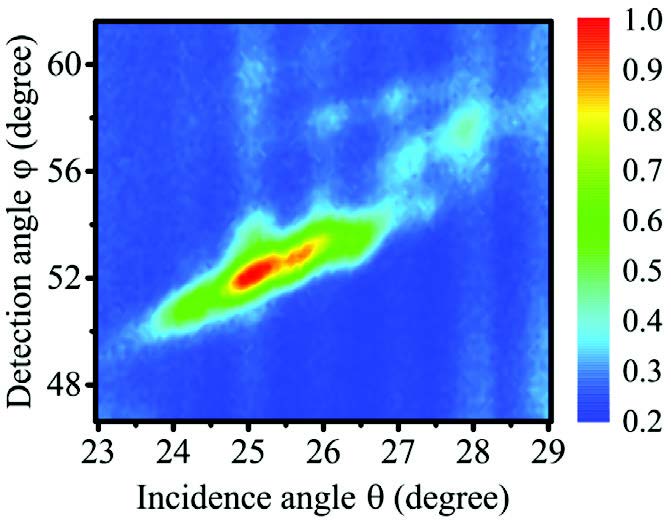
Fig. 1. Angular propagation of SH intensity depending on the angle of incidence of excitation radiation.
The indicatrix measured is shown on Fig. 2. An additional SH generation peak was found – not corresponding to the existing diffraction orders.
This peak is explained by the appearance of a periodic lattice in the PC which is directed across the layers. Such a lattice is formed due to the pendulum effect: certain beats arise between the modes of the PC, creating a periodic distribution of propagation that is stationary. Electro-induced quadratic sensitivity arises in peaks of this propagation.
The angular and spectral functions obtained confirm the synchronous character of SH generation. Applying Laue diffraction to the frequency conversion of light has more advantages as compared to using Bragg geometry, as the former allows one to use extended structures, and thus enhance the optical guide and effective length of interaction.
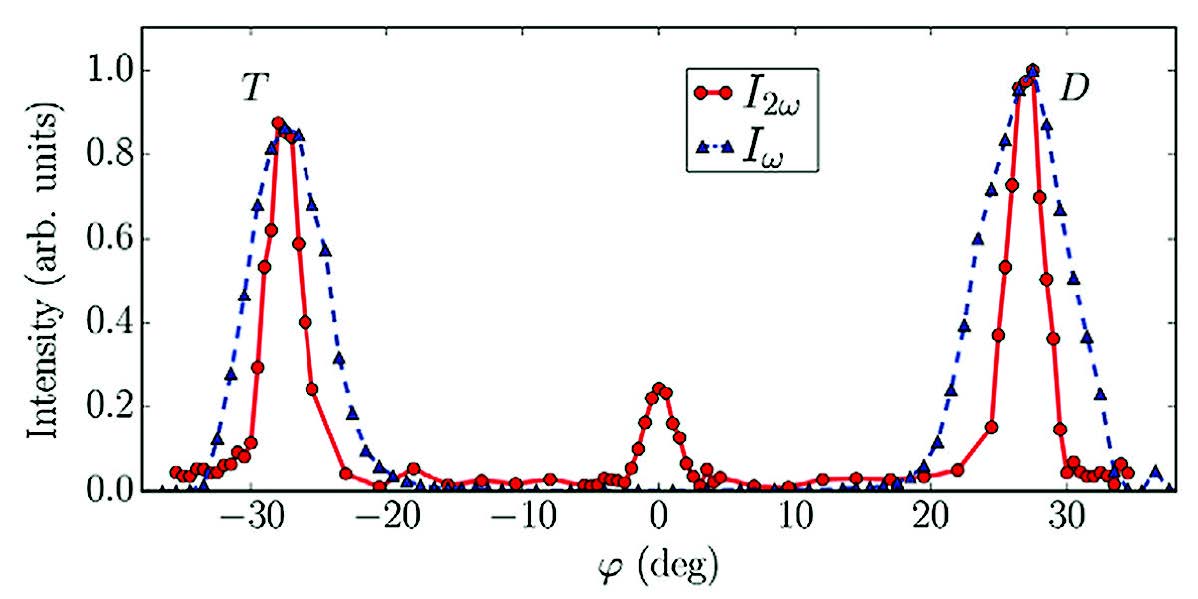
Fig. 2. Angular function of SH generation intensity in a crystal of silicon nanocrystallites.
D. A. Kopylov, S. E. Svyakhovskiy, L. V. Dergacheva, V. A. Bushuev, B. I. Mantsyzov, T. V. Murzina “Observation of optical second-harmonic generation in porous-silicon-based photonic crystals in the Laue diffraction scheme”, Physical Review A93, 053840 (2016);
V. B. Novikov, A. I. Maydykovskiy, B. I. Mantsyzov, T. V. Murzina “Laue diffraction in one-dimensional photonic crystals: The way for phase-matched secondharmonic generation.” Physical Review B93, 235420 (2016).